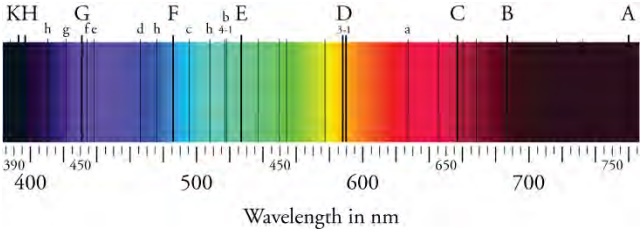
To tie the unique signatures of emission spectra to the composition of the atom itself would require clever thinking. Niels Bohr (1885-1962), a Danish physicist, did just that, by making immediate use of Rutherford's planetary model of the atom. Bohr, shown below, became convinced of its validity and spent part of 1912 at Rutherford's laboratory. In 1913, after returning to Copenhagen, he began publishing his theory of the simplest atom, hydrogen, based on Rutherford's planetary model.

Niels Bohr, Danish physicist, used the planetary model of the atom to explain the atomic spectrum and size of the hydrogen
atom. His many contributions to the development of atomic physics and quantum mechanics, his personal influence on many students and
colleagues, and his personal integrity, especially in the face of Nazi oppression, earned him a prominent place in history.
Bohr was able to derive the formula for the hydrogen spectrum using basic physics, the planetary model of the atom, and some very important new conjectures. His first conjecture was that only certain orbits are allowed: In other words, in an atom, the orbits of electrons are quantized. Each quantized orbit has a different distinct energy, and electrons can move to a higher orbit by absorbing energy or drop to a lower orbit by emitting energy. Because of the quantized orbits, the amount of energy emitted or absorbed must also be quantized, producing the discrete spectra seen in the image above. In equation form, the amount of energy absorbed or emitted can be found as
ΔE = Ei - Ef
where Ei refers to the energy of the initial quantized orbit, and refers to the energy of the final orbits. Furthermore, the wavelength emitted can be found using the equation
hf = Ei - Ef
and relating the wavelength to the frequency found using the equation v = fλ, where v corresponds to the speed of light.
It makes sense that energy is involved in changing orbits. For example, a burst of energy is required for a satellite to climb to a higher orbit. What is not expected is that atomic orbits should be quantized. Quantization is not observed for satellites or planets, which can have any orbit, given the proper energy (see below).
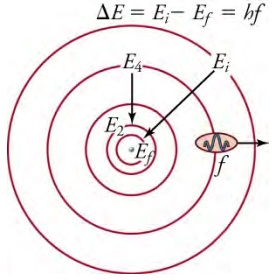
The planetary model of the atom, as modified by Bohr, has the orbits of the electrons quantized. Only certain orbits are
allowed, explaining why atomic spectra are discrete or quantized. The energy carried away from an atom by a photon comes from the
electron dropping from one allowed orbit to another and is thus quantized. The same is true for atomic absorption of photons.
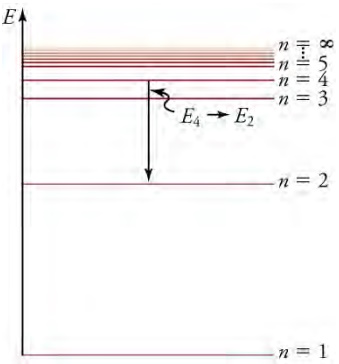
An-level diagram plots energy vertically and is useful in visualizing the energy states of a system and the transitions
between them. This diagram is for the hydrogen-atom electrons, showing a transition between two orbits having energies E4
and E2. The energy transition results in a Balmer series line in an emission spectrum.
The diagram above shows an energy-level diagram, a convenient way to display energy states. Each of the horizontal lines corresponds to the energy of an electron in a different orbital. Energy is plotted vertically with the lowest or ground state at the bottom and with excited states above. The vertical arrow downwards shows energy being emitted out of the atom due to an electron dropping from one excited state to another. That would correspond to a line shown on the atom's emission spectrum. The Lyman series shown in the spectrum results from electrons dropping to the ground state, while the Balmer and Paschen series result to electrons dropping to the n = 2 and n = 3 states, respectively.
Article source: OpenStax is a nonprofit educational technology initiative based at Rice University. OpenStax's mission is to improve educational access and learning for everyone. Textbooks on OpenStax's site are licensed under a Creative Commons Attribution 4.0 International License.

Learn more at amazon.com
